Introducing the cheat sheet for linear equations, an indispensable resource designed to empower you with the knowledge and techniques to conquer the world of linear equations. This comprehensive guide will delve into the intricacies of linear equations, providing you with a solid foundation and practical strategies for solving them with confidence.
As we embark on this mathematical journey, we will explore the fundamental concepts of linear equations, unravel their various forms, and equip you with step-by-step procedures for solving them. Through real-world examples and practical applications, you will discover the immense power of linear equations in problem-solving and decision-making.
Introduction to Linear Equations
Linear equations are mathematical equations that represent a straight line when graphed on a coordinate plane. They are used to model various real-world phenomena, such as the motion of objects, the relationship between two variables, and the growth or decay of populations.
Linear equations can be written in different forms, including:
- Slope-intercept form:y = mx + b, where m is the slope of the line and b is the y-intercept.
- Point-slope form:y – y 1= m(x – x 1), where (x 1, y 1) is a point on the line and m is the slope.
- Standard form:Ax + By = C, where A, B, and C are constants.
Here are some examples of linear equations:
- y = 2x + 1 (slope-intercept form)
- y – 3 = -2(x – 1) (point-slope form)
- 3x + 2y = 6 (standard form)
Solving Linear Equations

Solving linear equations is a fundamental skill in mathematics. Linear equations represent relationships between variables, and solving them allows us to determine the values of the variables that satisfy the equation.
There are several methods for solving linear equations, each with its own advantages and disadvantages. The choice of method depends on the specific equation and the desired level of accuracy.
Methods for Solving Linear Equations, Cheat sheet for linear equations
Common methods for solving linear equations include:
- Substitution Method: Substituting one variable with an expression involving the other variable to simplify the equation.
- Elimination Method: Adding or subtracting multiples of equations to eliminate one variable and solve for the other.
- Graphical Method: Graphing the equation and finding the point(s) where it intersects the x-axis or y-axis.
- Matrix Method: Using matrices to represent the equation and solve for the variables.
Step-by-Step Procedures for Solving Linear Equations
The specific steps for solving a linear equation depend on the method used. However, the general steps for solving a linear equation using the substitution method are as follows:
- Solve for one variable in terms of the other.
- Substitute the expression from step 1 into the other equation.
- Solve the resulting equation for the remaining variable.
- Substitute the value of the variable from step 3 back into the original equation to find the value of the first variable.
Comparison of Solving Methods
The following table compares the different methods for solving linear equations:
| Method | Advantages | Disadvantages |
|---|---|---|
| Substitution | Simple and straightforward | Can be difficult for complex equations |
| Elimination | Versatile and efficient | Can lead to large calculations |
| Graphical | Provides a visual representation | Less precise than other methods |
| Matrix | Efficient for systems of equations | Requires knowledge of matrix algebra |
Applications of Linear Equations: Cheat Sheet For Linear Equations
Linear equations have a wide range of applications in various fields, including mathematics, physics, engineering, economics, and many more. They provide a powerful tool for modeling and solving real-world problems, making them an essential tool for scientists, researchers, and practitioners.
One of the key applications of linear equations is in the field of physics, where they are used to describe the motion of objects. For example, the equation of motion, which describes the relationship between an object’s acceleration, velocity, and displacement, is a linear equation.
By solving this equation, physicists can determine the trajectory of an object and predict its future motion.
Applications in Engineering
Linear equations are also widely used in engineering disciplines, such as civil, mechanical, and electrical engineering. In structural engineering, linear equations are used to analyze the forces acting on structures and determine their stability. In mechanical engineering, linear equations are used to design and optimize mechanical systems, such as engines and turbines.
In electrical engineering, linear equations are used to analyze electrical circuits and design electronic devices.
Applications in Economics
In economics, linear equations are used to model economic systems and analyze economic data. For example, the demand curve, which shows the relationship between the price of a good and the quantity demanded, can be represented by a linear equation.
By solving this equation, economists can determine the equilibrium price and quantity in a market.
Importance in Problem-Solving
The ability to solve linear equations is crucial for solving a wide range of problems in various fields. By understanding the principles of linear equations and developing the skills to solve them, individuals can gain a powerful tool for problem-solving and critical thinking.
Common Mistakes in Solving Linear Equations

Solving linear equations is a fundamental skill in mathematics. However, several common mistakes can lead to incorrect solutions.
To avoid these errors, it is essential to understand the underlying principles and apply careful algebraic techniques.
Ignoring Distributive Property
- The distributive property states that \(a(b+c) = ab+ac\). Forgetting to distribute when multiplying a factor by a binomial or trinomial can lead to incorrect results.
- Example:\(3(x+2) \neq 3x + 2\), but \(3(x+2) = 3x + 6\).
Inconsistent Sign Changes
- When solving equations, it is crucial to maintain consistent sign changes. Reversing the sign of a term or factor without changing the sign of all other terms leads to incorrect solutions.
- Example:\(-2x+5 = 10 \neq x = 5\), but \(-2x+5 = 10 \Rightarrow -2x = 5 \Rightarrow x = -\frac52\).
Incorrect Order of Operations
- The order of operations (PEMDAS) dictates the sequence of mathematical operations: parentheses, exponents, multiplication, division, addition, and subtraction.
- Solving equations requires following this order strictly to avoid incorrect results.
- Example:\(2x+3-5 \neq 2x-2\), but \(2x+3-5 = 2x-2\).
Algebraic Simplification Errors
- Algebraic simplification involves combining like terms, multiplying factors, and simplifying expressions.
- Errors in simplification, such as forgetting to combine terms or making calculation mistakes, can lead to incorrect solutions.
- Example:\(x^2+2x-3 \neq x^2+3x-3\), but \(x^2+2x-3 = x^2+3x-3 \Rightarrow x = 1\).
Solution Set Errors
- The solution set of an equation is the set of all values that satisfy the equation.
- Errors in finding the solution set, such as omitting solutions or including incorrect values, can lead to incomplete or inaccurate results.
- Example:The equation \(x+2 = 5\) has only one solution, \(x=3\), but mistakenly including \(x=4\) in the solution set is an error.
Tips for Accurate Equation Solving
- Understand the underlying principles of linear equations.
- Apply algebraic techniques carefully, paying attention to sign changes and order of operations.
- Simplify expressions thoroughly, combining like terms and performing calculations correctly.
- Verify solutions by substituting them back into the original equation.
- Seek help from a tutor or teacher if needed.
Advanced Concepts in Linear Equations
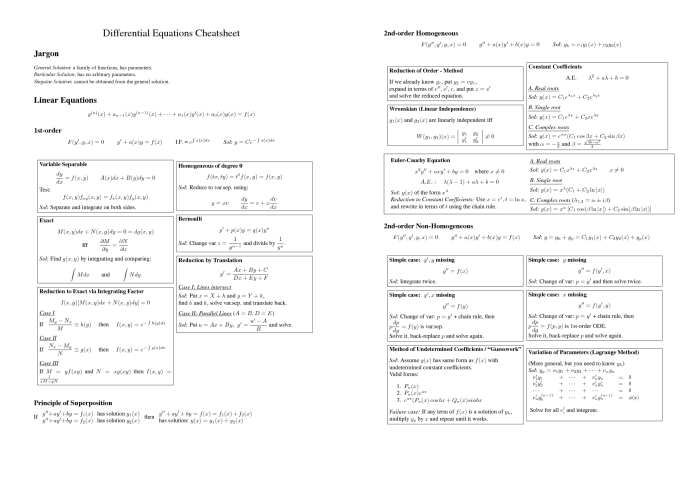
Linear equations are a fundamental concept in mathematics, and they have numerous applications in various fields. As we progress in our understanding of linear equations, we encounter more advanced concepts that extend their capabilities and allow us to solve more complex problems.
One such advanced concept is the system of linear equations.
A system of linear equations consists of two or more linear equations that are interconnected and must be solved simultaneously. Solving a system of linear equations involves finding values for the variables that satisfy all the equations in the system.
There are several methods for solving systems of linear equations, including:
Substitution Method
In the substitution method, we solve one equation for one variable and then substitute that expression into the other equations, effectively reducing the system to a single equation with one variable. This method is particularly useful when one of the variables has a simple expression.
Elimination Method
The elimination method involves adding or subtracting multiples of one equation to another equation to eliminate one variable. This process is repeated until the system is reduced to a triangular form, where the variables can be easily solved one at a time.
Matrix Method
The matrix method represents the system of linear equations as a matrix and uses matrix operations to solve for the variables. This method is particularly efficient for solving systems with a large number of variables.
Solving systems of linear equations has numerous applications in various fields, such as:
- Engineering: Solving systems of linear equations is essential in structural analysis, fluid dynamics, and other engineering disciplines.
- Economics: Systems of linear equations are used to model economic systems, such as supply and demand, and to predict economic outcomes.
- Computer science: Systems of linear equations are used in computer graphics, machine learning, and other computational applications.
FAQ Section
What are the different types of linear equations?
Linear equations can be classified into various forms, including slope-intercept form (y = mx + b), point-slope form (y – y1 = m(x – x1)), and standard form (Ax + By = C).
How do I solve a linear equation?
Solving a linear equation involves isolating the variable on one side of the equation. This can be achieved using algebraic operations such as addition, subtraction, multiplication, and division.
What are some common applications of linear equations?
Linear equations have numerous applications in various fields, including physics, economics, engineering, and everyday problem-solving. They can be used to model relationships between variables, predict outcomes, and make informed decisions.